

| Fixed-Point Blockset |   |
The IEEE Format
The IEEE Standard 754 has been widely adopted, and is used with virtually all floating-point processors and arithmetic coprocessors -- with the notable exception of many DSP floating-point processors.
Among other things, this standard specifies four floating-point number formats of which singles and doubles are the most widely used. Each format contains three components: a sign bit, a fraction field, and an exponent field. These components, as well as the specific formats for singles and doubles, are discussed below.
The Sign Bit
While two's complement is the preferred representation for signed fixed-point numbers, IEEE floating-point numbers use a sign/magnitude representation, where the sign bit is explicitly included in the word. Using this representation, a sign bit of 0 represents a positive number and a sign bit of 1 represents a negative number.
In general, floating-point numbers can be represented in many different ways by shifting the number to the left or right of the radix point and decreasing or increasing the exponent of the radix by a corresponding amount.
To simplify operations on these numbers, they are normalized in the IEEE format. A normalized binary number has a fraction of the form 1.f where f has a fixed size for a given data type. Since the leftmost fraction bit is always a 1, it is unnecessary to store this bit and is therefore implicit (or hidden). Thus, an n-bit fraction stores an n+1-bit number. The IEEE format also supports denormalized numbers, which have a fraction of the form 0.f. Normalized and denormalized formats are discussed in more detail in next section.
The Exponent Field
In the IEEE format, exponent representations are biased. This means a fixed value (the bias) is subtracted from the field to get the true exponent value. For example, if the exponent field is 8 bits, then the numbers 0 through 255 are represented, and there is a bias of 127. Note that some values of the exponent are reserved for flagging Inf (infinity), NaN (not-a-number), and denormalized numbers, so the true exponent values range from -126 to 127. See the sections Inf and NaN.
Single Precision Format
The IEEE single-precision floating-point format is a 32-bit word divided into a 1-bit sign indicator s, an 8-bit biased exponent e, and a 23-bit fraction f. A representation of this format is given below.
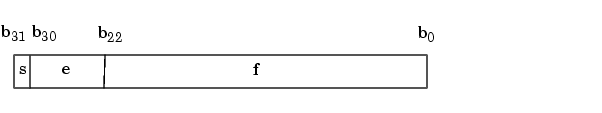
The relationship between this format and the representation of real numbers is given by
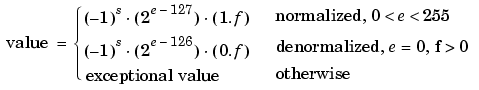
Exceptional Arithmetic discusses denormalized values.
Double Precision Format
The IEEE double-precision floating-point format is a 64-bit word divided into a 1-bit sign indicator s, an 11-bit biased exponent e, and a 52-bit fraction f. A representation of this format is given below.

The relationship between this format and the representation of real numbers is given by
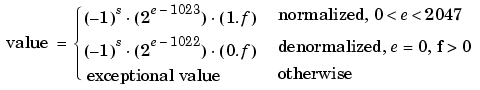
Exceptional Arithmetic discusses denormalized values.
Nonstandard IEEE Format
The Fixed-Point Blockset supports a nonstandard IEEE-style floating-point data type. This data type adheres to the definitions and formulas previously given for IEEE singles and doubles. You create nonstandard floating-point numbers with the float function:
TotalBits is the total word size and ExpBits is the size of the exponent field. The size of the fraction field and the bias are calculated from these input arguments. You can specify any number of exponent bits up to 11, and any number of total bits such that the fraction field is no more than 53 bits.
When specifying a nonstandard format, you should remember that the number of exponent bits largely determines the range of the result and the number of fraction bits largely determines the precision of the result.
 | Scientific Notation | Range and Precision |  |