Fixed-Point Blockset

Scientific Notation

A direct analogy exists between scientific notation and radix point notation. For example, scientific notation using five decimal digits for the fraction would take the form

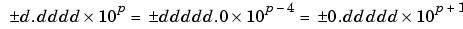
where p is an integer of unrestricted range. Radix point notation using five bits for the fraction is the same except for the number base

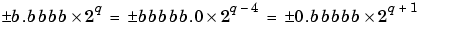
where q is an integer of unrestricted range. The previous equation is valid for both fixed- and floating-point numbers. For both these data types, the fraction can be changed at any time by the processor. However, for fixed- point numbers the exponent never changes, while for floating-point numbers the exponent can be changed any time by the processor.

For fixed-point numbers, the exponent is fixed but there is no reason why the radix point must be contiguous with the fraction. For example, a word consisting of three unsigned bits is usually represented in scientific notation in one of these four ways.

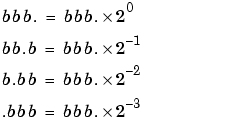
If the exponent were greater than 0 or less than -3, then the representation would involve lots of zeros.

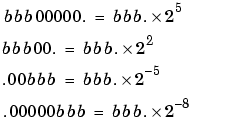
These extra zeros never change to ones, however, so they don't show up in the hardware. Furthermore, unlike floating-point exponents, a fixed-point exponent never shows up in the hardware, so fixed-point exponents are not limited by a finite number of bits.

Note    Restricting the radix point to being contiguous with the fraction is unnecessary; the Fixed-Point Blockset allows you to extend the radix point to any arbitrary location.

Floating-Point Numbers

The IEEE Format