Statistics Toolbox

Noncentral F Distribution

The following sections provide an overview of the noncentral F distribution.

Background of the Noncentral F Distribution

As with the 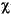² distribution, the F distribution is a special case of the noncentral F distribution. The F distribution is the result of taking the ratio of two 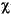² random variables each divided by its degrees of freedom.

If the numerator of the ratio is a noncentral chi-square random variable divided by its degrees of freedom, the resulting distribution is the noncentral F distribution.

The main application of the noncentral F distribution is to calculate the power of a hypothesis test relative to a particular alternative.

Definition of the Noncentral F Distribution

Similar to the noncentral 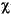² distribution, the toolbox calculates noncentral F distribution probabilities as a weighted sum of incomplete beta functions using Poisson probabilities as the weights.

I(x|a,b) is the incomplete beta function with parameters a and b, and 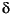is the noncentrality parameter.

Example and Plot of the Noncentral F Distribution

The following commands generate a plot of the noncentral F pdf.

• x = (0.01:0.1:10.01)';
  p1 = ncfpdf(x,5,20,10);
  p  = fpdf(x,5,20);
  plot(x,p,'--',x,p1,'-')
  
  
  

F Distribution

Gamma Distribution