Statistics Toolbox

Chi-Square Distribution

The following sections provide an overview of the 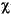² distribution.

Background of the Chi-Square Distribution

The 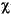² distribution is a special case of the gamma distribution where b = 2 in the equation for gamma distribution below.

The 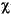² distribution gets special attention because of its importance in normal sampling theory. If a set of n observations is normally distributed with variance 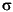², and s² is the sample standard deviation, then

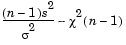
The Statistics Toolbox uses the above relationship to calculate confidence intervals for the estimate of the normal parameter 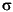² in the function normfit.

Definition of the Chi-Square Distribution

The ² pdf is

where ( · ) is the Gamma function, and is the degrees of freedom.

Example and Plot of the Chi-Square Distribution

The ² distribution is skewed to the right especially for few degrees of freedom (). The plot shows the ² distribution with four degrees of freedom.

• x = 0:0.2:15;
  y = chi2pdf(x,4);
  plot(x,y)
  
  
  

Binomial Distribution

Noncentral Chi-Square Distribution